| World!Of Numbers |  |
||
 The Nine Digits Page 4with some Ten Digits (pandigital) exceptions | |||
 Page 1 Page 2 Page 3 Page 5 Page 6 Page 7 | |||
When I use the term ninedigital in these articles I always refer to a strictly zeroless pandigital (digits from 1 to 9 each appearing just once).
Topic 4.5 [ July 4, 2005 ]
More Ninedigital Powers
by Peter Kogel
" Hi Patrick,
Herewith is another pandigital 'project' that I investigated some while ago.Notable results include:
213452 + 87962 = 532978641 The first term contains 1...5 and the second term contains 6...9.
25382 + 176492 = 29432 + 175862 = 317928645 Only example where two sums are equal.
5393 + 2463 + 7183 = 541623987
5393 + 4623 + 7183 = 625348179The above is what I call a 'pandigital amicable pair' (see below).
274 + 354 + 684 + 1494 = 516297843
274 + 354 + 864 + 1494 = 549617283Only one term on the left hand side is different.
95 + 175 + 265 + 435 + 585 = 816725493 Only example for 5th power.
Kind regards,
Pete Kogel. "
Pandigital diversions concerning 
a1P + a2P + ... + apP = N [ P ⩾ 2 ]
P = 2 A2 + B2 = N
E.g.:
In the above, the numbers A & B combined and also the result on the right hand side
contain the digits 1...9 (zero-less pandigital).
There are 78 such solutions ¬
A B | A B | A B | A B |
|---|---|---|---|
1596 24837 1635 29487 1875 24693 2367 19485 2436 18975 2538 17649 2784 15369 2943 17586 3495 12768 3516 28479 3654 12897 3846 29175 3864 25179 3915 28467 3984 26715 4197 25638 4359 16278 4395 27186 4536 12987 4698 21357 | 4857 21396 4896 21753 4965 17382 4968 15237 5367 14928 5376 12984 5376 18429 5436 17289 5469 13728 5496 18237 5613 27948 5679 14238 5697 21384 5763 21948 5964 18273 5976 21483 6189 25374 6198 23547 6345 21987 6453 19287 | 6459 17328 6471 23589 6471 29538 6528 13749 6549 27831 6813 24597 6831 29745 6945 13782 6975 14823 7164 25983 7356 24189 7359 28146 7431 29658 7458 23619 7659 13248 7659 13428 7824 19356 7863 21495 7869 23514 7953 16824 | 8259 13476 8295 14763 8349 17562 8352 17469 8517 29643 8745 16392 8793 12546 8796 21345 8796 23541 8934 15267 8973 12546 8976 25413 9156 27483 9168 23475 9627 18543 9687 24513 9735 24861 9741 26358 |
Observations
There are only 5 examples where A or B appears in more than one solution: viz ¬
There are several examples where simply swapping two digits in A leads to a member of another solution ¬
E.g.:
4395 5496 5697In the solution above, A contains the digits 1...5 and B contains the digits 6...9
The above is the only example where N is duplicated.
P = 3 A3 + B3 + C3 = N
E.g.:
A, B & C combined and N are zero-less pandigital.
There are 101 solutions; viz ¬
A B C | A B C | A B C | A B C |
|---|---|---|---|
123 465 897 124 389 576 124 635 789 124 639 875 126 593 784 128 634 759 129 375 468 129 365 847 132 479 865 135 297 684 136 582 749 137 286 954 138 259 746 138 264 795 139 576 824 142 396 857 145 297 863 145 296 873 145 693 728 146 397 825 147 265 983 149 258 637 153 469 728 156 492 738 159 423 768 162 354 789 | 165 324 789 165 234 978 167 532 894 172 348 596 175 629 843 176 549 832 178 426 593 182 379 564 184 527 936 186 395 742 189 246 537 192 548 763 192 356 847 193 468 572 194 285 736 194 376 825 194 627 835 196 273 458 196 342 785 197 364 825 198 243 675 213 467 589 213 578 649 215 378 496 216 397 485 216 387 495 | 219 438 567 219 436 587 231 456 789 234 619 857 237 654 819 246 539 718 249 381 675 251 486 739 253 419 768 254 391 768 258 314 697 259 641 837 261 457 938 267 384 951 269 537 841 271 398 546 271 458 639 274 591 863 278 351 496 279 413 856 312 479 658 317 489 526 318 547 692 321 498 576 321 564 897 328 519 674 | 342 596 871 351 468 792 362 459 718 372 519 864 374 526 918 378 512 649 381 469 572 386 519 724 394 561 728 397 458 621 398 415 672 418 693 725 429 576 831 429 671 835 462 539 718 483 597 621 487 521 693 489 613 725 493 581 627 493 512 786 514 639 782 574 639 812 591 624 738 |
While looking through the above list I noticed that some values occur in more than one solution
and I wondered whether I could chain several such solutions together; wherein the last number
becomes the first number in the next link: e.g. ¬
(123, 465, 897)
(897, 321, 564)
(564, 182, 379)
A slightly longer chain would be ¬
(193, 468, 572)
(572, 381, 469)
(469, 153, 728)
(728, 693, 145)
(145, 296, 873)
The longest chain that I found consists of 24 members ¬
(172, 348, 596) , (596, 871, 342) , (342, 785, 196) , (196, 273, 458) , (458, 271, 639) , (639, 875, 124) , (124, 389, 576) , (576, 831, 429) , (429, 671, 835) , (835, 194, 627) , (627, 581, 493) , (493, 786, 512) , (512, 649, 378) , (378, 215, 496) , (496, 278, 351) , (351, 792, 468) , (468, 193, 572) , (572, 381, 469) , (469, 153, 728) , (728, 145, 693) , (693, 418, 725) , (725, 613, 489) , (489, 317, 526) , (526, 918, 374)
This chain was found by 'hand' so it is possible that I may have missed a longer chain.
While searching for the above I came across the following nine-member 'pandigital sociable group'
i.e. the last member can be chained with the very first member.
 |
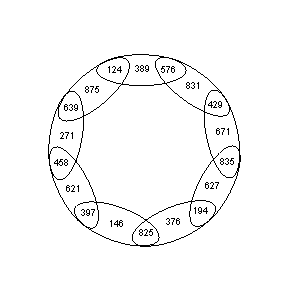(124, 389, 576) (576, 831, 429) (429, 671, 835) (835, 627, 194) (194, 376, 825) (825, 146, 397) (397, 621, 458) (458, 271, 639) (639, 875, 124) |
|---|
There is also one example of a 'pandigital amicable pair'.
(539, 246, 718)
(718, 462, 539)
Other notable curiosities(123, 564, 879) & (124, 639, 875) | | | | | | (231, 465, 897) & (142, 396, 857)The digits in A, B & C are permuted.
(231, 456, 789)B & C contain the series 456_789 in order.
(394, 561, 728) = (394, 394+167, 394+167+167)A, B, C are in arithmetic progression (difference = 167).
P = 4 A4 + B4 + C4 + D4 = N
E.g.:
A, B, C & D combined and N are zero-less pandigital.
There are only 5 solutions; viz ¬
The second and third solutions shown above are particularly noteworthy (another 'pandigital amicable pair').
P = 5 A5 + B5 + C5 + D5 + E5 = N
The above is the only solution.
P ⩾ 6 AP + BP + CP + DP + EP + FP + ... = N
No solutions.
Topic 4.4 [ March 24, 2005 ]
The Unique Pandigital 3816547290
by John Morse
" In the header of this message is 3,816,547,290 which is the only 10-digit
pandigital number whose first N digits are a multiple of N. I first discovered
that in The Dictionary of Curious and Interesting Numbers by David Wells,
and I wrote an explanation of how one could figure out that is the number.
John Morse
Albany New York USA "
Mathematics Puzzle
Find the one and only 10-digit number such that :
This explanation is my own, but I first learned about the amazing
mystery number (381,654,729) in an entertaining book, one of my ten
favorite math-themed books of all time :
Procedure
Let the letters A thru J stand for the digits of the number. Then :
A B C D E F G H I J is the mystery number, whereas :
AB is evenly divisible by 2,
ABC is evenly divisible by 3,
ABCD is evenly divisible by 4,
ABCDE is evenly divisible by 5,
ABCDEF is evenly divisible by 6,
ABCDEFG is evenly divisible by 7,
ABCDEFGH is evenly divisible by 8,
ABCDEFGHI is evenly divisible by 9,
ABCDEFGHIJ is evenly divisible by 10.
A number is evenly divisible by 10 only if its last digit is zero.
Hence, we have found right away that J must equal zero, since the
ten-digit number ends in that digit J.
What about divisibility by 9 ? This particular number takes care of that
matter because its nine-digit 'fragment' ABCDEFGHI must use one each of
digits 1 thru 9, and the sum of the nine digits is 45, which is a multiple of 9.
A number is evenly divisible by 9 if the sum of its digits is 9 or a
multiple of 9 itself. Hence, ANY arrangement of the digits 1 thru 9
(or 0 thru 9), each digit used exactly once, will always result in the
number being a multiple of 9.
A number is evenly divisible by 5 only if its last digit is a five or
a zero.
Since all the digits of ABCDEFGHIJ are different, we cannot use zero
since J already has that value.
The first five digits of the entire number are ABCDE, hence digit E
must be 5.
So far, the mystery number looks like this :
A B C D 5 F G H I 0
Even numbers only are evenly divisible by even divisors, e.g., if you
want to divide some number N by 2 or 4 or 6, etc., that number N must
be even since dividing an odd number by an even number will always leave
(an odd) remainder.
Hence, consider the mystery number's even-numbered digits. They
correspond to letters B, D, F, and H. Since zero is being used for J, the
even digits 2, 4, 6, and 8 must correspond to those other four letters.
Therefore, the remaining letters in the number - A, C, G, and I - must
stand for odd digits.
Because the odd digit 5 is already in use via the letter E, A can only
be 1, 3, 7, or 9. The same restrictions apply to C, G, and I.
There are many ways that AB is evenly divisible by 2 : merely let B
equal 2 or 4 or 6 or 8. Hence, there are too many possible ways so
far to assign digits for A and B. How about ABC, which must be a
multiple of 3 ?
The digits A + B + C must add up to a multiple of 3, and in this
instance, B is the only even digit. Hence, the following trios are possible
for fragment ABC :
Eighteen possible permutations exist for ABC; it looks like a lot of
trial and error lies ahead if we test all these 3-digit groups in
combination with the remaining digits for D, F, G, and H. (It does not
matter yet what digit I must be, since the entire 9-digit number up to I
is already divisible by 9.)
However, note that the four-digit fragment ABCD must be a multiple of 4,
and this is possible only if the number formed by the last two digits -
here, CD - is a multiple of 4.
Since C is odd, D can only be 2 or 6. If we try 4 or 8 instead for D, then we
get numbers such as 18, 34, 78, or 94 - none of which are evenly divisible by 4.
A similar situation arises for the 8-digit fragment ABCDEFGH. Since G
is odd, H can only be 2 or 6. A number is divisible by 8 only if its
last three digits (here, FGH) is evenly divisible by 8.
So, between them, D and H use up the digits 2 and 6. We are not sure
which letter will have which digit, but we can eliminate the 3-digit
fragments above where B happens to be 2 or 6, leaving these possible
permutations for the digit group ABC :
Good. Number of permutations is now only eight instead of eighteen.
Since B and F must be even, they must use the only remaining even
digits - 4 and 8 - between them. Let's take a look at the mystery
number where we assign the digits 2 and 6 to D and H and the digits
4 and 8 to B and F :
Consider the six-digit fragment ABCDEF. Its digits must add up to a multiple
of 3. All multiples of six are even, but we already know that F must be even.
Since the digits ABC add up to a multiple of 3, so must those in fragment
DEF. Since E is 5, only two possibilities exist for digits in DEF :
So, we can eliminate two of the four 10-digit numbers above, leaving :
Let's take another look at the 8-digit fragment ABCDEFGH and see if we
can determine whether F must be 4 or 8.
If D=2, then B must be 4, F must be 8, and H must be 6. Since FGH must
be a multiple of 8, it could only end in the following three digits :
Of these four 3-digit numbers, 836 and 876 are NOT multiples of 8, so
eliminate them, leaving only 816 and 896 for FGH.
If D=6, then B must be 8, F must be 4, and H must be 2. Since FGH must
be a multiple of 8, it could only end in the following three digits :
Of these four 3-digit numbers, 412 and 492 are NOT multiples of 8, so
eliminate them, leaving only 432 and 472 for FGH.
Hence, possible arrangements of digits in the mystery number could be :
Recall that fragment ABC must be a multiple of 3. In choice [1], the
only available digits for A and C are 3, 7, and 9. No combination of
these digits will enable ABC to be a multiple of 3, so eliminate choice [1].
For choice [2], the only digits available are 1, 3, and 9. No combination
of these will make ABC a multiple of 3, so scratch choice [2].
For choice [3], the only digits left are 1, 7, and 9. The only workable
combination that will make ABC a multiple of 3 is when neither A nor C
is 7, so I must be 7.
For choice [4], the only digits available are 1, 3, and 9. Digits
1 and 3 may represent A and C, in which case I must be 9. If digits
1 and 9 stand in for A and C, then I must be 3.
So, two choices for the mystery number were removed, leaving these
possible configurations for the mystery number :
Almost there! Each of these six numbers is such that the number AB is
divisible by 2, ABC by 3, ABCD by 4, ABCDE by 5, ABCDEF by 6, ABCDEFGH
by 8, ABCDEFGHI by 9, and ABCDEFGHIJ by 10.
What about the fragment ABCDEFG ? Is it divisible by 7 ? Test each of
the six numbers above using their first seven digits :
[1] 1896543 divided by 7 leaves remainder 5. nope.
[2] 9816543 divided by 7 leaves remainder 2. nope.
[3] 1836547 divided by 7 leaves remainder 6. nope.
[4] 3816547 divided by 7 leaves remainder 0. YES !
[5] 1896547 divided by 7 leaves remainder 2. nope.
[6] 9816547 divided by 7 leaves remainder 6. nope.
Aha! Only choice [4] leaves no remainder when its fragment ABCDEFG is
divided by 7.
Finally we found the mystery number ! It is
| 3 8 1 6 5 4 7 2 9 0 |
Are all the digits different ? Yes. A number with that property is
known as "pandigital". The prefix "pan-" means "all".
How about divisibility of the first N digits by N ? Check and see :
38 divided by 2 = 19, no remainder. Yawn.
381 divided by 3 = 127, no remainder. Okay.
3816 divided by 4 = 954, no remainder. Okay!
38165 divided by 5 = 7633, no remainder. Big deal.
381654 divided by 6 = 63609, no remainder. Good.
3816547 divided by 7 = 545221, no remainder. Good!
38165472 divided by 8 = 4770684, no remainder. Great!
381654729 divided by 9 = 42406081, no remainder. Of course.
3816547290 divided by 10 = 381654729, no remainder. Wonderful!!!
There is no other 10-digit number whose first N digits are evenly
divisible by N - and where every digit occurs once !
Other internet sources discussing this topic :
http://ken.duisenberg.com/potw/archive/arch96/960919sol.html
http://www.nrich.maths.org.uk/public/viewer.php?obj_id=796&part=solution&refpage=viewer.php
http://begghilos2.ath.cx/~jyseto/Academia/Math-Problem-2.php
http://www.rodoval.com/heureka/probsnum.html
... many more when 3816547290 is entered as keyword in GOOGLE for instance !
Topic 4.3 [ February 27, 2005 ]
Nine and Ten digit squares
by Peter Kogel
" I've had a fascination for numbers and rec maths ever since I first read
Martin Gardner's column in Scientific American many years ago. My particular
fascination is for nine and ten digit number patterns and I've found your
excellent website the source of much inspiration.This project has been to investigate patterns along the lines of 99066^2
( 9814072356 ) and have found some interesting results that you might like.Some highlights
77772277772 = 60485271895340361729 The square contains each digit 0...9 twice and the square root is a rather
particular palindrome.
42539071862 = 1809572634_7102438596 The lefthand side is pandigital and the square on the righthand side contains
the digits 0...9 repeated in each half (there are two other such examples).
Nine and Ten digit squares
It is well known that the square of 11826 ( 139854276 ) contains all the digits 1...9
and that the square of 32043 ( 1026753849 ) contains all the digits 0...9.
Others have investigated cases where the digits in the square are repeated more than once.
I have extended this investigation up to the 6th repeat of the digits. My findings are shown below.
| N | D | Comments |
|---|---|---|
| 11826 [ A071519 ] 30384 335180136 10546200195312 333350001269641272 10540978243301566001337 |
1 1 2 3 4 5 |
Smallest Largest Smallest Smallest Smallest Smallest |
| Where D = The number of times the 9 digits (1...9) are repeated in N squared | ||
| N | D | Comments |
|---|---|---|
| 32043 [ A054038 ] 99066 3164252736 316245509988426 31622952459028694643 3162279417919838932896672 > 9.9999994 x 10^29 |
1 1 2 3 4 5 6 |
Smallest Largest Smallest Smallest Smallest Smallest Largest |
| Where D = The number of times the 10 digits (0...9) are repeated in N squared | ||
I haven't yet found the largest example where all ten digits are repeated 6 times.
Perhaps someone else can find it and perhaps extend the table even further.
While searching for the above I came across the following interesting pair:
A and B differ by only two digits!
Pandigital squares
It is well known that the squares of A = 57321 and B = 60984 each contain all ten digits
and that 'A & B' combined also contains the ten digits. I wondered whether such results
could be extended such that 'A & B' contain the digits repeated twice. I very soon found
literally hundreds of solutions of which the following type is of interest:
Note that the squares of 'A' & 'B' each contain all ten digits repeated twice,
'A' contains no digit larger than 5 and 'A & B' combined contains all ten digits repeated twice.
There are many other such examples.
There are also many examples where 'A' and 'B' are pan-digital. E.g.:
Similarly the following are pan-9-digital
While searching for these examples I came across the following remarkable trio:
Notice that the digits 0...9 are contained in each 'half' of the square
on the right hand side. There are no pan-9-digital equivalents.
[ Tom Marlow discovered only the first two squares. He submitted it to Ed Pegg's
Mathpuzzle site. Source 
material added 26 January 2003.]
Complete listing of the above squared nine- and pandigitals
with the higher powers as well,
up to the fifth for ninedigitals
up to the sixth for pandigitals
available at https://web.archive.org/web/20040909153834/http://blue.kakiko.com/mmrmmr/htm/eqtn11.html
Palindromes
There are many examples where N is a palindrome.
I have not found any solutions where the square is also a palindrome
and I suspect that none exist.
Higher powers
It is natural to ask whether the above results can be extended to higher powers.
The answer is of course "yes!"
E.g.
In the following, the notation (N, P, D) means that the digits 1...9 (or 0...9)
are repeated 'D' times in the value of NP.
Nine digit examples:
| (N, 3, 2) has 2 solutions | N = 496536, 982617 |
| (N, 4, 2) has 1 solution | N = 24267 |
| (N, 4, 3) has 8 solutions | N = 3374532, 3928791, 4143474, 4552878, 4714896, 4796571, 4905006, 5577408 |
| (N, 5, 4) has 2 solutions | N = 12036828, 12343788 |
Ten digit examples: [ A074205 ]
| (N, 3, 2) has 138 solutions | N = 2158479, 2190762, 2205528, 2219322, ... ..., 4631793 |
| (N, 4, 2) has 4 solutions | N = 69636, 70215, 77058, 80892 |
| (N, 5, 3) has 7 solutions | N = 643905, 680061, 720558, 775113, 840501, 878613, 984927 |
| (N, 6, 4) has ? solutions | N = 3470187, ... Source: OEIS |
| (N, 7, 4) has 2 solutions | N = 421359, 493107 |
| (N, 8, 5) has ? solutions | N = 1472157, ... Source: OEIS |
| (N, 9, 5) has 1 solution | N = 320127 |
| (N, 9, 6) has 1 solution | N = 3976581 |
| (N, 10, 8) has ? solutions | N = 81785058, ... ..., 95927037 |
| (N, 11, 8) has 1 solution | N = 15763347 |
| (N, 12, 9) has 1 solution | N = 31064268 |
| (N, 13,10) has 2 solutions | N = 44626422, 44695491 |
| (N, 14, 12) has 2 solutions | N = 330096453, 346527657 |
| (N, 15, 12) has 1 solution | N = 85810806 |
The challenge above was to make 'D' as small as possible for any particular 'P'.
I also have constructed a table of smallest and largest of any particular type
up to and including P = 15.
There are also examples where P = D in viz:
Nine digit examples:
| (481514667, 3, 3) Smallest | (998782725, 3, 3) Largest |
| (590291892, 4, 4) Smallest | (998709318, 4, 4) Largest |
| (653813013, 5, 5) Smallest | (989543343, 5, 5) Largest |
| (853836318, 6, 6) Smallest | (897702789, 6, 6) Largest |
I have not yet found any example where P = D = 7 and I suspect there are none
because it becomes increasingly unlikely that the power of any number will not
contain a zero when P is large.
Ten digit examples:
| (4642110594, 3, 3) Smallest | (9999257781, 3, 3) Largest |
| (5623720662, 4, 4) Smallest | (9999112926, 4, 4) Largest |
| (6312942339, 5, 5) Smallest | (9995722269, 5, 5) Largest |
| (6813614229, 6, 6) Smallest | (9999409158, 6, 6) Largest |
| (7197035958, 7, 7) Smallest | (9998033316, 7, 7) Largest |
| (7513755246, 8, 8) Smallest | (9993870774, 8, 8) Largest |
| (7747685775, 9, 9) Smallest | (9986053188, 9, 9) Largest |
| (7961085846, 10, 10) Smallest | (9964052493, 10, 10) Largest |
| (8120306331, 11, 11) Smallest | (9975246786, 11, 11) Largest |
| (8275283289, 12, 12) Smallest | (9966918135, 12, 12) Largest |
| (8393900487, 13, 13) Smallest | (9938689137, 13, 13) Largest |
| (8626922994, 14, 14) Smallest | (9998781633, 14, 14) Largest |
| (8594070624, 15, 15) Smallest | (9813743148, 15, 15) Largest |
I have not checked any power larger than 15 as yet.
Pandigital powers
There are a few examples where 'N' is pandigital.
Complete listing available at https://web.archive.org/web/20040909153834/http://blue.kakiko.com/mmrmmr/htm/eqtn11.html
Nine digit examples:
| (345918672, 2, 2) Smallest | (976825431, 2, 2) Largest | # 28 |
345918672^2 = 119659727638243584 | ||
| (516473892, 3, 3) Smallest | (751396842, 3, 3) Largest | # 5 |
| (N, 4, 4) No solutions | # 0 | |
| (961527834, 5, 5) only 1 solution | # 1 | |
Ten digit examples:
| (3175462089, 2, 2) Smallest | (9876124053, 2, 2) Largest | # 534 |
| (4680215379, 3, 3) Smallest | (9863527104, 3, 3) Largest | # 74 |
| (5702631489, 4, 4) Smallest | (9846032571, 4, 4) Largest | # 13 |
| (7351062489, 5, 5) Smallest | (9847103256, 5, 5) Largest | # 8 |
| (7025869314, 6, 6) Smallest | (9247560381, 6, 6) Largest | # 6 |
There do not appear to be any examples for higher powers.
A curious pan-digital example is the following
Notice that the left hand side including the index contains the digits 0...9 and that the right hand side
contains the digits 1...9 repeated 3 times. I have found no other such example for any power.
Palindromes
To date I haven't searched for palindrome solutions for cubes and higher powers
but I have no reason to believe that they do not exist. I'll investigate further when
I have a bit more time.
Topic 4.2 [ May 18, 2005 ]
Nine and Ten digit powers
More results from Peter Kogel
Here are some more results from Peter Kogel which can be added as an addendum :
333333500000125024109149626 squared, contains the digits 1..9 repeated six times (smallest) 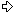111111222222333349447774470014236868657845737074239129
999999944444387333120727861 squared, contains the digits 1..9 repeated six times (largest) 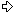999999888888777752667554514316632321351744642421635321
3162277835852223520386730813562 contains digits 0..9 repeated six times (smallest) 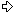100000011111222223236955438883649634757986945845567794798736
9999999444443873456035583500562 contains digits 0..9 repeated six times (largest) 999999888888777777633214105446041204560215564562321035203136
32541967082 = 10589_7962143580_37264 digits 0..9 contained in the centre and each digit repeated twice overall
85916740232 = 73816_8625174930_04529 ditto
89520631742 = 80139_4350712869_54276 ditto
90761483522 = 82376_4689075123_15904 ditto
96831047522 = 93762_5176382049_81504 ditto
97058431262 = 94203_3907865214_51876 ditto
43259073 = 80952_7368052419_17643 digits 0..9 contained in the centre (only cubic example)
869122976116 contains the digits 0..9 repeated sixteen times (smallest)
997090225216 contains the digits 0..9 repeated sixteen times (largest)
880038967817 contains the digits 0..9 repeated seventeen times (smallest)
974038376717 contains the digits 0..9 repeated seventeen times (largest)
998581978520 =
972018388122736146908354492278598571766247280628254566407260946047011341
594668735384194513571360871003364967097249794743843109249267940157739156
83917180391558815561723889985220630315208530426025390625
Ie. the 200 digit number contains each digit 0..9 repeated 20 times!
This is the largest such example.
" I used Yuji Kida's excellent UBASIC for all my calculations. The
program I wrote is not very elegant (I am only an amateur programmer)
and the algorithm I developed is probably far from optimal. Indeed,
from what I have learned from this project I would probably tackle it
from a completely different angle if I were ever to do it again. "
Topic 4.1 [ December 29, 2004 ]
Solutions for ABC + DEF = GHI
Linda Rojewski
Linda Rojewski asked for all the combinations of a three digit number added
to a three digit number to equal a three digit number. You can only use digits 1-9,
and can not use any number twice. You can add to carry over a number of which
this number would not count as usage of that number.
Solution:
I (pdg) used the well known UBASIC tool and the result is listed below.
In total there are 336 solutions though not all are unique!
124 + 659 = 783 is listed as well as 659 + 124 = 783.
Question:
From this list it should be easy to extract only the distinct solutions.
How many do you count ?
Send them in and I will highlight them.
1 124659783 124 + 659 = 783 2 125739864 125 + 739 = 864 3 127359486 127 + 359 = 486 4 127368495 127 + 368 = 495 5 128367495 128 + 367 = 495 6 128439567 128 + 439 = 567 7 129357486 129 + 357 = 486 8 129438567 129 + 438 = 567 9 129654783 129 + 654 = 783 10 129735864 129 + 735 = 864 11 134658792 134 + 658 = 792 12 135729864 135 + 729 = 864 13 138429567 138 + 429 = 567 14 138654792 138 + 654 = 792 15 139428567 139 + 428 = 567 16 139725864 139 + 725 = 864 17 142596738 142 + 596 = 738 18 142695837 142 + 695 = 837 19 143586729 143 + 586 = 729 20 145692837 145 + 692 = 837 21 146583729 146 + 583 = 729 22 146592738 146 + 592 = 738 23 152487639 152 + 487 = 639 24 152784936 152 + 784 = 936 25 154629783 154 + 629 = 783 26 154638792 154 + 638 = 792 27 154782936 154 + 782 = 936 28 157329486 157 + 329 = 486 29 157482639 157 + 482 = 639 30 158634792 158 + 634 = 792 31 159327486 159 + 327 = 486 32 159624783 159 + 624 = 783 33 162387549 162 + 387 = 549 34 162783945 162 + 783 = 945 35 163782945 163 + 782 = 945 36 167328495 167 + 328 = 495 37 167382549 167 + 382 = 549 38 168327495 168 + 327 = 495 39 173286459 173 + 286 = 459 40 173295468 173 + 295 = 468 41 175293468 175 + 293 = 468 42 176283459 176 + 283 = 459 43 182367549 182 + 367 = 549 44 182394576 182 + 394 = 576 45 182457639 182 + 457 = 639 46 182493675 182 + 493 = 675 47 182754936 182 + 754 = 936 48 182763945 182 + 763 = 945 49 183276459 183 + 276 = 459 50 183492675 183 + 492 = 675 51 183546729 183 + 546 = 729 52 183762945 183 + 762 = 945 53 184392576 184 + 392 = 576 54 184752936 184 + 752 = 936 55 186273459 186 + 273 = 459 56 186543729 186 + 543 = 729 57 187362549 187 + 362 = 549 58 187452639 187 + 452 = 639 59 192384576 192 + 384 = 576 60 192483675 192 + 483 = 675 61 192546738 192 + 546 = 738 62 192645837 192 + 645 = 837 63 193275468 193 + 275 = 468 64 193482675 193 + 482 = 675 65 194382576 194 + 382 = 576 66 195273468 195 + 273 = 468 67 195642837 195 + 642 = 837 68 196542738 196 + 542 = 738 69 214569783 214 + 569 = 783 70 214659873 214 + 659 = 873 71 215478693 215 + 478 = 693 72 215748963 215 + 748 = 963 73 216378594 216 + 378 = 594 74 216738954 216 + 738 = 954 75 218349567 218 + 349 = 567 76 218376594 218 + 376 = 594 77 218439657 218 + 439 = 657 78 218475693 218 + 475 = 693 79 218736954 218 + 736 = 954 80 218745963 218 + 745 = 963 81 219348567 219 + 348 = 567 82 219438657 219 + 438 = 657 83 219564783 219 + 564 = 783 84 219654873 219 + 654 = 873 85 234657891 234 + 657 = 891 86 235746981 235 + 746 = 981 87 236718954 236 + 718 = 954 88 236745981 236 + 745 = 981 89 237654891 237 + 654 = 891 90 238419657 238 + 419 = 657 91 238716954 238 + 716 = 954 92 239418657 239 + 418 = 657 93 241596837 241 + 596 = 837 94 243576819 243 + 576 = 819 95 243675918 243 + 675 = 918 96 245673918 245 + 673 = 918 97 245718963 245 + 718 = 963 98 245736981 245 + 736 = 981 99 246573819 246 + 573 = 819 100 246591837 246 + 591 = 837 101 246735981 246 + 735 = 981 102 248319567 248 + 319 = 567 103 248715963 248 + 715 = 963 104 249318567 249 + 318 = 567 105 251397648 251 + 397 = 648 106 254619873 254 + 619 = 873 107 254637891 254 + 637 = 891 108 257391648 257 + 391 = 648 109 257634891 257 + 634 = 891 110 259614873 259 + 614 = 873 111 264519783 264 + 519 = 783 112 269514783 269 + 514 = 783 |
113 271593864 271 + 593 = 864 114 271683954 271 + 683 = 954 115 273186459 273 + 186 = 459 116 273195468 273 + 195 = 468 117 273546819 273 + 546 = 819 118 273591864 273 + 591 = 864 119 273645918 273 + 645 = 918 120 273681954 273 + 681 = 954 121 275193468 275 + 193 = 468 122 275418693 275 + 418 = 693 123 275643918 275 + 643 = 918 124 276183459 276 + 183 = 459 125 276318594 276 + 318 = 594 126 276543819 276 + 543 = 819 127 278316594 278 + 316 = 594 128 278415693 278 + 415 = 693 129 281394675 281 + 394 = 675 130 281673954 281 + 673 = 954 131 283176459 283 + 176 = 459 132 283671954 283 + 671 = 954 133 284391675 284 + 391 = 675 134 286173459 286 + 173 = 459 135 291357648 291 + 357 = 648 136 291384675 291 + 384 = 675 137 291546837 291 + 546 = 837 138 291573864 291 + 573 = 864 139 293175468 293 + 175 = 468 140 293571864 293 + 571 = 864 141 294381675 294 + 381 = 675 142 295173468 295 + 173 = 468 143 296541837 296 + 541 = 837 144 297351648 297 + 351 = 648 145 314658972 314 + 658 = 972 146 316278594 316 + 278 = 594 147 317529846 317 + 529 = 846 148 317628945 317 + 628 = 945 149 318249567 318 + 249 = 567 150 318276594 318 + 276 = 594 151 318627945 318 + 627 = 945 152 318654972 318 + 654 = 972 153 319248567 319 + 248 = 567 154 319527846 319 + 527 = 846 155 324567891 324 + 567 = 891 156 324657981 324 + 657 = 981 157 327159486 327 + 159 = 486 158 327168495 327 + 168 = 495 159 327519846 327 + 519 = 846 160 327564891 327 + 564 = 891 161 327618945 327 + 618 = 945 162 327654981 327 + 654 = 981 163 328167495 328 + 167 = 495 164 328617945 328 + 617 = 945 165 329157486 329 + 157 = 486 166 329517846 329 + 517 = 846 167 341586927 341 + 586 = 927 168 342576918 342 + 576 = 918 169 346572918 346 + 572 = 918 170 346581927 346 + 581 = 927 171 348219567 348 + 219 = 567 172 349218567 349 + 218 = 567 173 351297648 351 + 297 = 648 174 352467819 352 + 467 = 819 175 354618972 354 + 618 = 972 176 354627981 354 + 627 = 981 177 357129486 357 + 129 = 486 178 357291648 357 + 291 = 648 179 357462819 357 + 462 = 819 180 357624981 357 + 624 = 981 181 358614972 358 + 614 = 972 182 359127486 359 + 127 = 486 183 362187549 362 + 187 = 549 184 362457819 362 + 457 = 819 185 364527891 364 + 527 = 891 186 367128495 367 + 128 = 495 187 367182549 367 + 182 = 549 188 367452819 367 + 452 = 819 189 367524891 367 + 524 = 891 190 368127495 368 + 127 = 495 191 372546918 372 + 546 = 918 192 376218594 376 + 218 = 594 193 376542918 376 + 542 = 918 194 378216594 378 + 216 = 594 195 381294675 381 + 294 = 675 196 381546927 381 + 546 = 927 197 382167549 382 + 167 = 549 198 382194576 382 + 194 = 576 199 384192576 384 + 192 = 576 200 384291675 384 + 291 = 675 201 386541927 386 + 541 = 927 202 387162549 387 + 162 = 549 203 391257648 391 + 257 = 648 204 391284675 391 + 284 = 675 205 392184576 392 + 184 = 576 206 394182576 394 + 182 = 576 207 394281675 394 + 281 = 675 208 397251648 397 + 251 = 648 209 415278693 415 + 278 = 693 210 418239657 418 + 239 = 657 211 418275693 418 + 275 = 693 212 419238657 419 + 238 = 657 213 428139567 428 + 139 = 567 214 429138567 429 + 138 = 567 215 438129567 438 + 129 = 567 216 438219657 438 + 219 = 657 217 439128567 439 + 128 = 567 218 439218657 439 + 218 = 657 219 452187639 452 + 187 = 639 220 452367819 452 + 367 = 819 221 457182639 457 + 182 = 639 222 457362819 457 + 362 = 819 223 462357819 462 + 357 = 819 224 467352819 467 + 352 = 819 |
225 475218693 475 + 218 = 693 226 478215693 478 + 215 = 693 227 482157639 482 + 157 = 639 228 482193675 482 + 193 = 675 229 483192675 483 + 192 = 675 230 487152639 487 + 152 = 639 231 492183675 492 + 183 = 675 232 493182675 493 + 182 = 675 233 514269783 514 + 269 = 783 234 517329846 517 + 329 = 846 235 519264783 519 + 264 = 783 236 519327846 519 + 327 = 846 237 524367891 524 + 367 = 891 238 527319846 527 + 319 = 846 239 527364891 527 + 364 = 891 240 529317846 529 + 317 = 846 241 541296837 541 + 296 = 837 242 541386927 541 + 386 = 927 243 542196738 542 + 196 = 738 244 542376918 542 + 376 = 918 245 543186729 543 + 186 = 729 246 543276819 543 + 276 = 819 247 546183729 546 + 183 = 729 248 546192738 546 + 192 = 738 249 546273819 546 + 273 = 819 250 546291837 546 + 291 = 837 251 546372918 546 + 372 = 918 252 546381927 546 + 381 = 927 253 564219783 564 + 219 = 783 254 564327891 564 + 327 = 891 255 567324891 567 + 324 = 891 256 569214783 569 + 214 = 783 257 571293864 571 + 293 = 864 258 572346918 572 + 346 = 918 259 573246819 573 + 246 = 819 260 573291864 573 + 291 = 864 261 576243819 576 + 243 = 819 262 576342918 576 + 342 = 918 263 581346927 581 + 346 = 927 264 583146729 583 + 146 = 729 265 586143729 586 + 143 = 729 266 586341927 586 + 341 = 927 267 591246837 591 + 246 = 837 268 591273864 591 + 273 = 864 269 592146738 592 + 146 = 738 270 593271864 593 + 271 = 864 271 596142738 596 + 142 = 738 272 596241837 596 + 241 = 837 273 614259873 614 + 259 = 873 274 614358972 614 + 358 = 972 275 617328945 617 + 328 = 945 276 618327945 618 + 327 = 945 277 618354972 618 + 354 = 972 278 619254873 619 + 254 = 873 279 624159783 624 + 159 = 783 280 624357981 624 + 357 = 981 281 627318945 627 + 318 = 945 282 627354981 627 + 354 = 981 283 628317945 628 + 317 = 945 284 629154783 629 + 154 = 783 285 634158792 634 + 158 = 792 286 634257891 634 + 257 = 891 287 637254891 637 + 254 = 891 288 638154792 638 + 154 = 792 289 642195837 642 + 195 = 837 290 643275918 643 + 275 = 918 291 645192837 645 + 192 = 837 292 645273918 645 + 273 = 918 293 654129783 654 + 129 = 783 294 654138792 654 + 138 = 792 295 654219873 654 + 219 = 873 296 654237891 654 + 237 = 891 297 654318972 654 + 318 = 972 298 654327981 654 + 327 = 981 299 657234891 657 + 234 = 891 300 657324981 657 + 324 = 981 301 658134792 658 + 134 = 792 302 658314972 658 + 314 = 972 303 659124783 659 + 124 = 783 304 659214873 659 + 214 = 873 305 671283954 671 + 283 = 954 306 673245918 673 + 245 = 918 307 673281954 673 + 281 = 954 308 675243918 675 + 243 = 918 309 681273954 681 + 273 = 954 310 683271954 683 + 271 = 954 311 692145837 692 + 145 = 837 312 695142837 695 + 142 = 837 313 715248963 715 + 248 = 963 314 716238954 716 + 238 = 954 315 718236954 718 + 236 = 954 316 718245963 718 + 245 = 963 317 725139864 725 + 139 = 864 318 729135864 729 + 135 = 864 319 735129864 735 + 129 = 864 320 735246981 735 + 246 = 981 321 736218954 736 + 218 = 954 322 736245981 736 + 245 = 981 323 738216954 738 + 216 = 954 324 739125864 739 + 125 = 864 325 745218963 745 + 218 = 963 326 745236981 745 + 236 = 981 327 746235981 746 + 235 = 981 328 748215963 748 + 215 = 963 329 752184936 752 + 184 = 936 330 754182936 754 + 182 = 936 331 762183945 762 + 183 = 945 332 763182945 763 + 182 = 945 333 782154936 782 + 154 = 936 334 782163945 782 + 163 = 945 335 783162945 783 + 162 = 945 336 784152936 784 + 152 = 936 |
Topic 4.5 → 213458796, 253817649, 294317586, 539246718, 539462718, 273568149, 273586149, 917264358
P=2 → 159624837, 163529487, 187524693, 236719485, 243618975, 253817649, 278415369, 294317586, 349512768, 351628479, 365412897, 384629175, 386425179, 391528467, 398426715, 419725638, 435916278, 439527186, 453612987, 469821357, 485721396, 489621753, 496517382, 496815237, 536714928, 537612984, 537618429, 543617289, 546913728, 549618237, 561327948, 567914238, 569721384, 576321948, 596418273, 597621483, 618925374, 619823547, 634521987, 645319287, 645917328, 647123589, 647129538, 652813749, 654927831, 681324597, 683129745, 694513782, 697514823, 716425983, 735624189, 735928146, 743129658, 745823619, 765913248, 765913428, 782419356, 786321495, 786923514, 795316824, 825913476, 829514763, 834917562, 835217469, 851729643, 874516392, 879312546, 879621345, 879623541, 893415267, 897312546, 897625413, 915627483, 916823475, 962718543, 968724513, 973524861, 974126358
P=3 → 123465897, 124389576, 124635789, 124639875, 126593784, 128634759, 129375468, 129365847, 132479865, 135297684, 136582749, 137286954, 138259746, 138264795, 139576824, 142396857, 145297863, 145296873, 145693728, 146397825, 147265983, 149258637, 153469728, 156492738, 159423768, 162354789, 165324789, 165234978, 167532894, 172348596, 175629843, 176549832, 178426593, 182379564, 184527936, 186395742, 189246537, 192548763, 192356847, 193468572, 194285736, 194376825, 194627835, 196273458, 196342785, 197364825, 198243675, 213467589, 213578649, 215378496, 216397485, 216387495, 219438567, 219436587, 231456789, 234619857, 237654819, 246539718, 249381675, 251486739, 253419768, 254391768, 258314697, 259641837, 261457938, 267384951, 269537841, 271398546, 271458639, 274591863, 278351496, 279413856, 312479658, 317489526, 318547692, 321498576, 321564897, 328519674, 342596871, 351468792, 362459718, 372519864, 374526918, 378512649, 381469572, 386519724, 394561728, 397458621, 398415672, 418693725, 429576831, 429671835, 462539718, 483597621, 487521693, 489613725, 493581627, 493512786, 514639782, 574639812, 591624738
P=4 → 246978135, 273568149, 273586149, 485796123, 647285139
P=5 → 917264358
Linda Rojewski (email) - go to topic
Peter Kogel (email) - go to topic - go to topic addendum - go to more ninedigital powers
John Morse (email) - go to topic
[
TOP OF PAGE]
( © All rights reserved ) - Last modified : September 3, 2024.
Patrick De Geest - Belgium
- Short Bio - Some PicturesE-mail address : pdg@worldofnumbers.com